Abstract
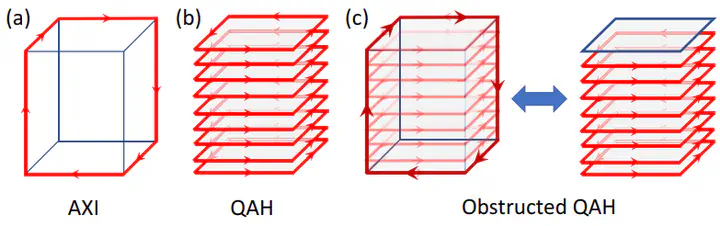
In recent theoretical and experimental investigations, researchers have linked the low-energy field theory of a Weyl semimetal gapped with a charge-density wave (CDW) to high-energy theories with axion electrodynamics. However, it remains an open question whether a lattice regularization of the dynamical Weyl-CDW is in fact a single-particle axion insulator (AXI). In this Rapid Communication, we use analytic and numerical methods to study both lattice-commensurate and incommensurate minimal (magnetic) Weyl-CDW phases in the mean-field state. We observe that, as previously predicted from field theory, the two inversion (I)-symmetric Weyl-CDWs with ϕ=0,π differ by a topological axion angle δθϕ=π. However, we crucially discover that neither of the minimal Weyl-CDW phases at ϕ=0,π is individually an AXI; they are instead quantum anomalous Hall (QAH) and “obstructed” QAH insulators that differ by a fractional translation in the modulated cell, analogous to the two phases of the Su-Schrieffer-Heeger model of polyacetylene. Using symmetry indicators of band topology and non-Abelian Berry phase, we demonstrate that our results generalize to multiband systems with only two Weyl fermions, establishing that minimal Weyl-CDWs unavoidably carry nontrivial Chern numbers that prevent the observation of a static magnetoelectric response. We discuss the experimental implications of our findings and provide models and analysis generalizing our results to nonmagnetic Weyl- and Dirac-CDWs.